Real Numbers Properties of Real Numbers For any real numbers a, b and c; $$ \forall \; a, b \in R \quad \Rightarrow \quad a+b \in R $$ $$ \forall \; a, b \in R \quad \Rightarrow \quad a . b \in R $$ $$ (a + b) + c = a + (b +…
Row Matrix A matrix is called a row matrix, if it has only one row. e.g., the matrix $ M = \begin {bmatrix} 2 & -1 & 7 \\ \end{bmatrix} $ is a row matrix of order 1-by-3 and $ N = \begin {bmatrix} 1 & -1 \\ \end{bmatrix} $ is a row matrix of…
Question 1. Find the order of the following matrices. Solution: $$ A = \begin {bmatrix} 2 & 3 \\ -5 & 6 \\ \end{bmatrix} $$ Order of matrix A is 2-by-2 $$ B = \begin {bmatrix} 2 & 0 \\ 3 & 5 \\ \end{bmatrix} $$ Order of matrix B is 2-by-2 $$…
Question 1. Write the following quadratic equations in the standard form and point out pure quadratic equations. $ \pmb{ (i). \;\;\;\;\; (x + 7) (x − 3) = -7} $ Solution: The given quadratic equation is $$ (x + 7) (x − 3) = -7 \;\;\;\;\; (i) $$ Multiplying the expressions $ (x + 7)…
In this method, write the quadratic equation in the standard form as ax2 + bx + c = 0 (i) If two numbers r and s can be found for the equation (i) such that r + s = b and rs = ac then ax2 + bx + c can be factorized into two…
The matrices and determinants are used in the field of Mathematics, Physics, Statistics, Electronics and other branches of science. The matrices have played a very important role in this age of computer science. The idea of matrices was given by Arthur Cayley, an English mathematician of nineteenth century, who first developed, “Theory of Matrices” in…
To solve a quadratic equation by the method of completing square is illustrated through the following examples. Example Solve the equation x2 − 3x − 4 = 0 by completing square. Solution: x2 − 3x − 4 = 0 (i) Shifting constant term −4 to the right, we have x2 − 3x = 4 …
To find the solution set of a quadratic equation, following methods are used: factorization completing square use of quadratic formula
An equation of 2nd degree is called quadratic equation. In more detail, the quadratic equation is an equation, which contains the square of the unknown (variable) quantity, but no higher power. General or standard form of a quadratic equation: A 2nd degree equation in one variable x of the form as below, where a ≠ 0…
A set is a collection of well defined distinct objects or symbols. Well defined objects mean that the objects possess a property that enables one to determine whether a given object is in the collection or not. For example, a set cannot consist of elements like moral values, concepts, evils or virtues. Element of a…
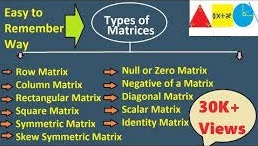
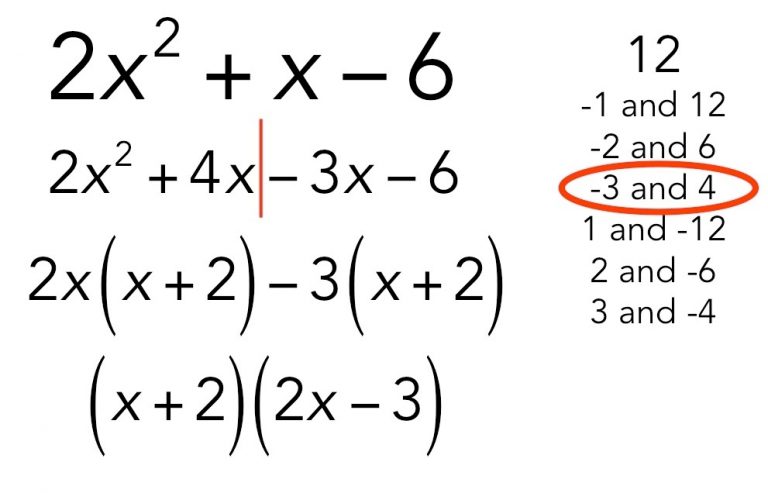
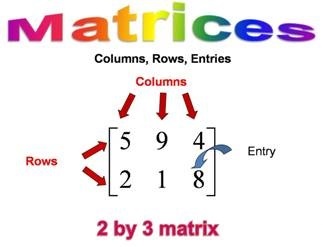
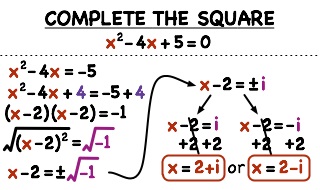
You must be logged in to post a comment.